Relativity visualized
In the rest frame of the bicycle the hubs are at rest and the wheels rotate in stationary motion (Figure 11a). In the rest frame of the street the wheels execute a combination of translation and rotation. A measurement of the shape of such a rolling wheel reveals the distorted shape shown in Figure 11b. The rim of the rolling wheel moves fastest at the top where it is thus most strongly length contracted so that the spokes come closer together. At the point of support the rim of the tyre is momentarily at rest and thus not length contracted, the spokes are therefore farther apart than in the wheel in stationary rotation.
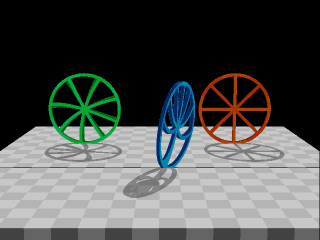
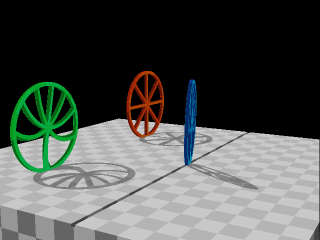
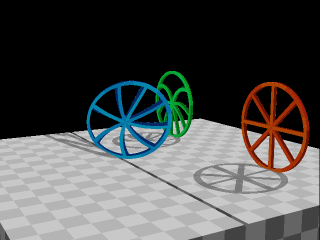
When rotating and rolling wheels are watched, the measured distortions are combined with the effects of the light travel times as described above. All of them can be detected in the three images of Figure 12. These images show the same scene from three different viewpoints. Three wheels can be seen, one of them at rest (red), one in stationary clockwise rotation (green) and one that rolls in a flat track from left to right (blue).
When the view on the scene is from the front (Fikgure 12a) so that the viewing direction is approximately perpendicular to the planes of the wheels, then the effects of light travel times are small since the light travel time to the camera is approximately the same from all points on the wheels. One therefore essentially sees the same shapes that would be measured (Figure 11). The rolling wheel appears rotated - as expected - and also a little tilted to the front. The reason for this is that our view onto the wheel is a little oblique.
When following the rolling wheel with the eye (Figure 12b), it is conspicuous that from this viewing direction the spokes of the green wheel in stationary rotation also are closer together at the top than at the bottom. This distortion is purely an effect of the light travel times: the top part of the wheel approaches the observer and appears lengthened, the bottom one recedes and appears contracted.
When looking towards the rolling wheel (Figure 12c), the distortion of the wheel in stationary rotation just turns around. The spokes of the rolling wheel appear nearly undistorted because the effects of length contraction (spokes closer together at the top) and light travel times (top part of the wheel approaches the observer and appears stretched) nearly cancel in the projected image.
Contact: Would you like to send us a message?