Relativity visualized
Gamow's cyclist rides at 93% of the speed of light as can easily be inferred from the length contraction of the wheels in Figure 1. For the motion of a single wheel this means: The point on the wheel momentarily in contact with the street is at rest, the hub moves at v=0.93c (c the speed of light) and the point on the rim on top of the wheel moves at 0.93c with respect to the hub, i.e. with respect to the street at 2v/(1+v2/c2) = 0,997c according to the relativistic velocity addition.
However, if one wants to set a wheel from rest into rotation at nearly the speed of light, a serious mechanical problem arises: The rim that moves in the direction of its circumference will be length contracted, at a rim speed of v=0.93c by a factor of γ=2,7. But the motion of the spokes is perpendicular to their axes so that the spokes are not shortened.
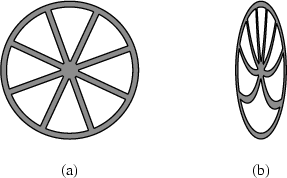
Without going into technical details we therefore equip the bicycle with wheels that are assembled in rotation. This is done in such a way that in stationary rotation the wheels have the geometric shape of ordinary wheels at rest (Figure 11a).
An ant living on the rim of the wheel would then measure a circumference that is not π times but 8.5 times the diameter of the wheel: the intrinsic geometry of the wheel defined in this way is not Euclidean [ 9].
Contact: Would you like to send us a message?
,
Corvin Zahn,
Date: May 25, 2002